Introduction.

In the following I shall show that there is an intimate - holistic - context between microcosmos - the atomic world - and macrocosmos - the Universe as a whole. I shall deduct equations allowing us to calculate cosmological quantities as the age, extension and total mass of the Universe by means of atomic physical quantities and Newton's gravitational 'constant'. The only quantity, which has not yet been measured with great accuracy, is the extension of the electron, but it is supposed to be of the order smaller than 10^—18 m. The deducted equations are based on my discovery of two equations — # (1) and (2) — establishing connections between physical quantities from microcosmos, f.i. Planck's Constant, the mass of an electron, its extension and electrical charge, and quantities characteristical for the Universe as a whole, such as its mass, extension and age, and furthermore the velocity of light and Newton's gravitational constant. The discovered equations form part of my quantum cosmology, with the main title: 'Holistic Quantum Cosmology with Decreasing Gravity'. The treatise is found on the Internet at the URL: http://louis.rostra.dk/.

The cosmologic basic equation

As my equation, given by (1), gives a context between quantities characterizing microcosmos and quantities characterizing macrocosmos I call it for the cosmologic basic equation:

(1)

In equation (1) R is the actual extension of the Universe, k_C is Coulomb's Constant, e is the electric charge of the electron, m_eits mass, G Newton's gravitational 'constant', h Planck's Constant, c₀ is the velocity of light and M₀ is the total matter/energy of the Universe. The fraction in the first parenthesis, denominated N = 4.16 · 10⁴², gives the ratio between the electrostatic forces and the gravitostatic forces between two electrons. The ratio between Planck's Constant and the product of the total mass of the Universe and the velocity of light gives the physical smallest distance in the Universe and is therefore called the elementary length.
The value of R is - also in the established cosmology - determined to be of the order 10 ²⁶ m and M₀ is in my quantum cosmology calculated to be about 1.6 · 10⁶⁰ kg, a value I calculate based on a 'measured' relative variation of Newton's gravitational 'constant'.

The ratio between the mass density of the electron and the mass density of the Universe.

Let us calculate the ratio between the mass density, , of an electron and the average mass density of the Universe, :

(2)

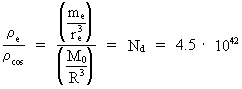
In (2) the following values are used: R = 10²⁶ m ; r_e =0.5·10^—18 m ; m_e = 9.11·10^—31 kg; M₀ = 1.6·10⁶⁰ kg.
We see very interestingly that N_d is very near to the value of N. This can not be accidental!!! If more exact values are used, I am sure that N_d will be found to be equal to N. That a context exists between the average density of the Universe, the density of the electron and N is a logical consequence of the evolution of the Universe from a smaller and more dense Universe to a bigger and less dense Universe. The quantity N is not a constant, but plays a role as a cosmic evolution quantum number, which had a much smaller value when electrons were created in the young Universe. Electrons - and thereby all matter - are 'remnants' from the earliest Universe, which have not yet been dissolved. (see the above URL). Assuming that N_d = N, we see that a context exists between the quantities of equation (1) and the quantities of equation (2). The discovery of this context is certainly found by means of some known values of R and M₀, but on the other hand, by means of this context R and M₀ can be calculated from atomic physical quantities and Newton's gravitaional 'constant'.

Calculation of the extension, age and mass of the Universe.

Combining (1) and (2) we can calculate the extension, R, of the Universe by the following equation:

(3)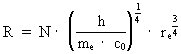

If we for the extension of the electron, r_e use the value 0.5·10^—18 m we get for for R:

(4)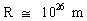

which of course is not surprising.

R can also be written as:

(5)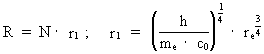

where r₁ gives a characteristic length: r₁ = 2.4 · 10^–17 m
The age, T, of the Universe can be calculated by the equation:

(6)

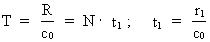
where t₁ gives a characteristic time interval: t₁ = 7.8 · 10^–26 s.
The quantity h/(m_e·c₀) is the ’Compton wavelength’ of the electron.
The mass of the Universe, M₀, can be calculated by the following equation:

(7)

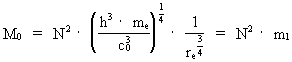
where m₁ is a characteristic mass: m₁ = 9.2 · 10^–26 kg.

Decreasing gravity and the increasing electron.

From the deducted equations it can be seen that with an expanding Universe, where R is increasing, one or more of the used quantities must vary, gradually as the Universe expands. The question is: Which of the quantities varies? There are many indications that G decreases as the Universe expands. This is treated in my quantum cosmology, where it is also assumed that the mass of the Universe is kept constant. As it is also assumed that the mass of the electron is constant, this leads to the observation that the extension of the electron must vary, gradually as the Universe expands. The calculations show (see above reference) that the extension of the electron increases. In other words: The electron is growing! For the temporal relative increase of the extension of the electron the following is valid:

(8)

where T is the actual age of the Universe. We see from equation (8) that the increase of the electron is extremely small in our epoch, about 8.5·10^–11 per year. In earlier epochs of the Universe the increase has been faster. In other words, the electron has been smaller when the Universe was younger. This also seems to be a logical evolution, for how could there otherwise be space enough for electrons in the very earliest and extremely much smaller Universe? If you ask: does the extension of protons and other composite particles also vary as the Universe expands? Then the reply must be Yes!

© Louis Nielsen, 20. nov. 1997
E-mail: LNi@Herlufsholm.dk

  Next article